Dynamics of Flow Networks in Porous Media

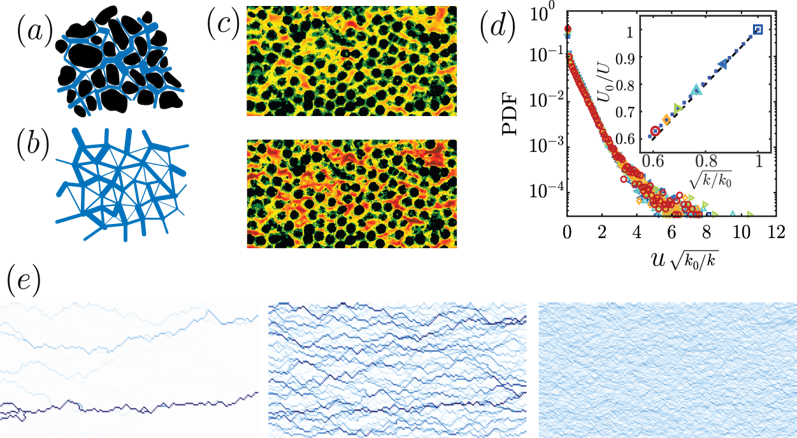
Pore-level flow velocity magnitude illustrations

Pore-level flow velocity magnitude: (a) Cross-section of a porous media (sandstone) obtained using computerized tomography scan; (b) Schematic of a topologically random network; (c) Experimental results of fluid velocity in a porous media made of glass-beads before (top-row) and after polymer flow (bottom row) which results in changes in network structure; (d) Probability density function, PDF, of the magnitude of flow velocities normalized by the inverse square root of the permeability. Inset shows the linear relation between relative average velocity and the inverse square root of permeability; (e) different long-time behavior of a porous network depending on various erosion laws, changing from channeling instability (left) to homogenization (right) indicating the rich physics in a porous network system. (manuscript under preparation)

Understanding flow and transport of fluids and material in porous media is important for many environmental applications. A team at the Harvard MRSEC led by Amir and Weitz have modelled porous media as a network of pores connected with cylindrical tubes representing the pore-throats. Using this simplified model, they explain the experimentally observed exponential distribution of local fluid velocity in a disordered porous media composed of random packing of glass beads. Their model considers the evolution of systems that either become clogged (permeability decrease over time) or eroded (permeability increases over time). They find rich dynamics in which the flow networks either exhibit increasing heterogeneity (clogging regime) or become more homogeneous (erosion regime) over time.

Ariel Amir (Applied Math), and
David A. Weitz (Physics & Applied Physics)
2020-2021 Harvard MRSEC (DMR-2011754)